In the previous article we have discussed the Carnot cycle, and its efficiency. But the Carnot cycle has a low mean effective pressure. To increase the mean effective pressure, theoretically achieve the maximum efficiency, there are some modifications can be done to the Carnot cycle. This modified Carnot cycle is called the Stirling Cycle.
Stirling Cycle
In this Stirling Cycle, there are two Isothermal processes and two Isochoric processes (constant volume processes).
The P-V diagram and T-S diagrams for the Stirling Cycle shown below.
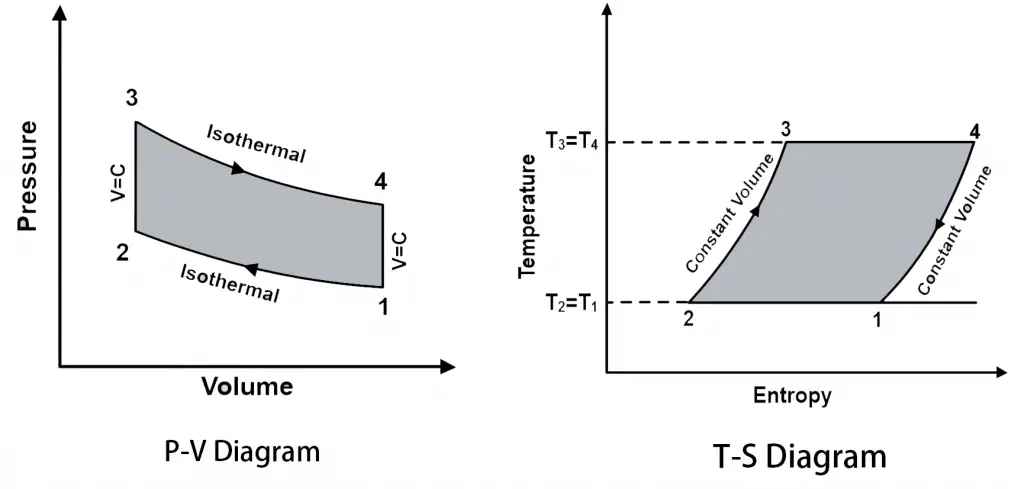
The different processes in the Stirling cycle.
- 3 → 4 (Isothermal Expansion)
- 4 → 1 (Isochoric Expansion)
- 1 → 2 (Isothermal Compression)
- 2 → 3 (Isochoric Compression)
The only difference is that during the processes 4 → 1 and the 2 → 3, instead of the adiabatic processes, they have made it as the constant volume processes (Isochoric).
From the above T-S diagram of the Stirling cycle, it is observed that during the constant volume processes, the heat added to the system from the source and the heat rejected to sink both are same. (i.e T3 = T4 and T2 = T1 respectively).
Click here to Check the Carnot cycle processes
The efficiency of the Stirling Cycle is
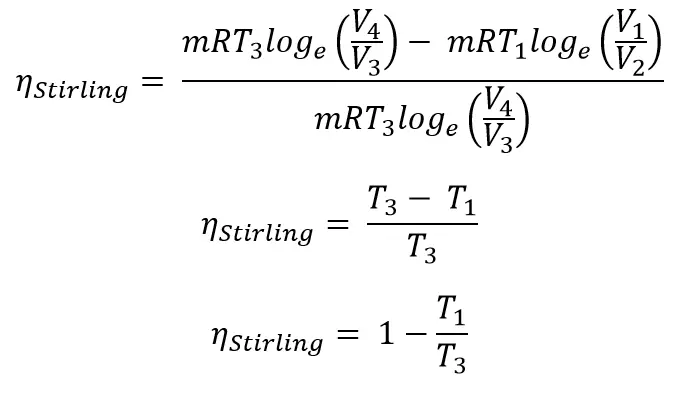
The efficiency of the Stirling cycle is as same as the Carnot cycle efficiency.
Practically it is not that easy to build a Heat Exchanger to operate continuously at that much high temperatures. This is the major difficulty in the Stirling Engines. So these became obsolete after the introduction of an Otto cycle and the diesel Cycles.
Conclusion
We have discussed the Stirling cycle of how it is different from the Carnot cycle. Please let us know your thoughts in the comment section below.

Leave a Reply