Natural Frequency of Free Torsional Vibrations

In the previous articles, we have discussed how to find the Natural Frequency for Free Longitudinal Vibrations and for free Transverse Vibrations. In this article, we are going to discuss the Natural Frequency of Free Torsional Vibrations.

As we know the natural Frequency of the Free Torsional Vibrations can be determined by the following methods.

1. Equilibrium Method
2. Energy Method
3. Rayleigh’s method

All these three methods are discussed in detail in the previous article. But for now, we will determine the natural frequency of torsional vibrations with the help of the Equilibrium method only.

Equilibrium Method for determining Natural frequency of free Torsional Vibrations

Let’s consider a shaft whose one end is fixed and on the other hand, is attached to a body of weight W as shown in the below figure.

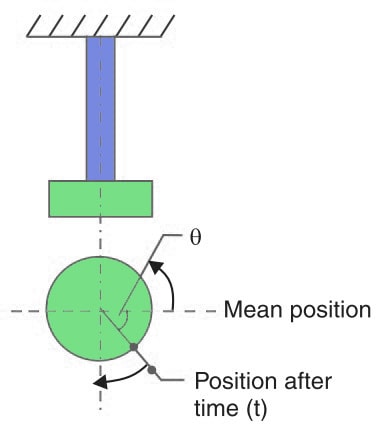
Where

• θ = Angular displacement of the shaft from the mean position after time t in radians
• m = Mass of the disc in kg
• I = Mass moment of inertia of disc in kg-m² = m.k²
• k = Radius of gyration in metres
• q = Torsional stiffness of the shaft in N-m

Now, let’s see the body is in the equilibrium position,

∴ Restoring force = q.θ

The accelerating force

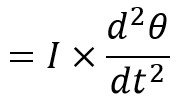
From the above two equations of motion of the body of mass m after time t is given by

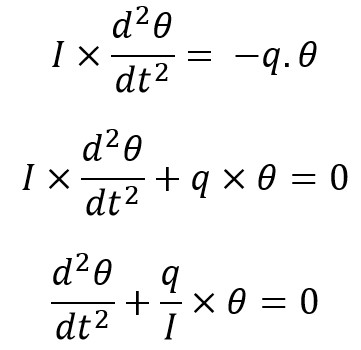
From the fundamental equation of the simple harmonic motion of the body is

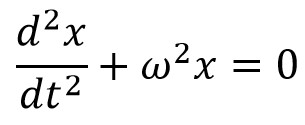
So equating these two similar equations will get us

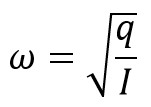
∴ Time period

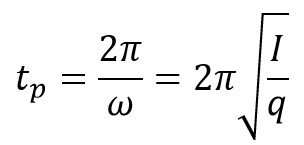
The natural frequency

The torsional stiffness q can be calculated from the torsion equation.

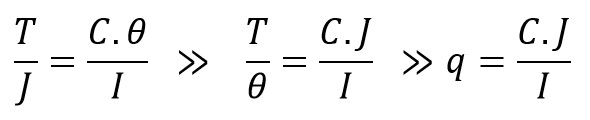
(∴ q= T/θ)

Where

• T =Torque
• θ = Twist
• C = Modulus of rigidity for the shaft material
• J = Polar moment of inertia of the shaft cross-section (πd⁴ /32) where d is the diameter of the shaft
• l = Length of the shaft

Conclusion

We have derived the equation for finding the natural frequency of the Free Torsional Vibrations with Equilibrium Method. The Energy Method, Rayleigh’s methods you can try if you wanted to by taking the previous article. If you have any further thoughts on this topic, let us know in the comment section below.