With the first article, on the Theory of machines, we have discussed the definitions for the Kinematics, kinetics, dynamics and statics. In this article, we are going to discuss the kinematics of motion. which will cover the study of the displacement, velocity and acceleration in both linear and angular directions.
Kinematics of Motion
Kinematics of motion deals with the relative motion between the various parts of the machines without considering the forces causing the motion. Simply the kinematics deals with the geometry of motion and concepts like displacement, velocity and acceleration with respect to the time.
The displacement, velocity and acceleration will be having different definitions when they are in the linear motion and angular motion. Let’s discuss them with the definitions and their units.
Linear Displacement
The distance moved by an object with respect to a fixed point is said as the linear displacement. This displacement can be along a straight line or a curved path.

This linear displacement is a vector quantity. It has both magnitude and direction.
The S.I units for linear displacement is a meter(m)
Linear Velocity
The rate of change at which an object moving from one point to another with respect to the time is called the Linear velocity.
Simply the rate of change of linear displacement of an object with respect to the time said as the linear velocity.

The Linear Velocity is also a vector quantity. It has both a magnitude and a direction.
Mathematically, the linear velocity v = ds/dt
The S.I Units for the linear velocity is meter per second (m/s)
Are Linear velocity and speed are the same?
The speed is the rate of change of linear displacement of a body with respect to the time. Since the speed is irrespective of its direction, therefore, it is a scalar quantity. It should not be confused with the linear velocity.
Linear Acceleration
The rate of change of linear velocity of an object with respect to the time said as the linear acceleration.
The Linear Acceleration is also a vector quantity. It has both a magnitude and a direction.
Mathematically, the linear Acceleration a = dv/dt
The S.I Units for the linear velocity is Meter per second2 (m/s2)
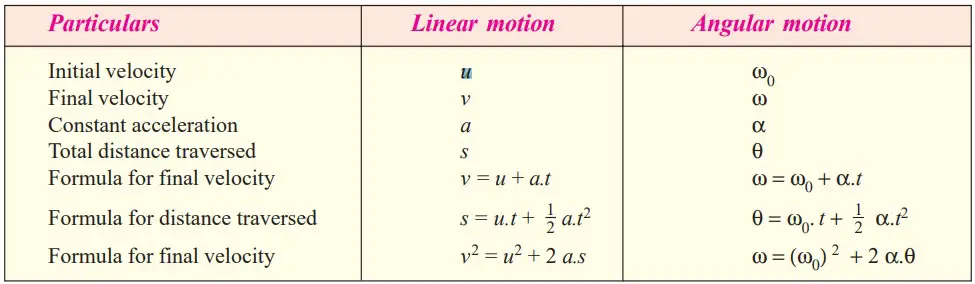
Equations of the Linear Motion
Following are the important linear motion equations to understand in the theory of machine subject point of view.
- v = u + a.t
- s = u.t + 1/2 a.t2
- v2 = u2 + 2a.s
- s = vav × t
- u = Initial velocity of the body
- v = Final velocity of the body
- a = Acceleration of the body
- s = Displacement of the body in time t seconds
- vav = Average velocity of the body during the motion (u+v)/2
Important things to keep in mind to use the above equations
- In case of the vertical motion of the object the acceleration, a will be acceleration due to the earth gravity which will be represented by the g.
- The value of g is taken as +9.81 m/s2 for downward motion, -9.81 m/s2 for the upward motion.
- When an object is falling freely from a height h then the velocity can be given as v2 = 2gh
Angular Displacement
It is defined as the angular distance moved by an object from one point to another.
This angular displacement is a vector quantity. It has both magnitude and direction.
The S.I units for angular displacement is degrees
Angular velocity
It is an angular distance moved by an object from one point to another with respect to the time.
Simply the rate of change of angular displacement with respect to the time.
The angular velocity is denoted by ω (omega)
Mathematically, the angular velocity is represented by ω= dθ/dt
The S.I Units for the angular velocity is rad/sec
Are the angular velocity and rotational speed are the same?
The rotational speed is a measure of the rotations per minute (RPM) whereas the angular velocity is the measure of the angle with respect to the time along with the direction. So Angular velocity is a vector quantity, whereas the speed is a scalar quantity. since it does not have the direction.
If the direction of angular velocity is constant, then the rate of change of magnitude of angular displacement with respect to the time is termed as the angular speed.
Angular Acceleration
The rate of change of angular velocity of an object with respect to the time said as the angular acceleration.
The angular acceleration denoted by α (alpha)
The angular Acceleration is also a vector quantity. It has both a magnitude and a direction.
Mathematically, the linear Acceleration α = dω/dt
The S.I Units for the linear velocity is rad/sec2
Equations of Angular Motion
Following are the important angular motion equations to understand in the theory of machine subject point of view.
- ω= ω0 +α.t
- θ = ω0t + 1/2 α.t2
- ω2 = (ω0)2 +2α.θ
- θ = 1/2(ω+ω0)t
- ω0 = Initial angular velocity in rad/s
- ω = Final angular velocity in rad/s
- t = Time in seconds
- θ = Angular displacement in time t seconds
- α = Angular acceleration in rad/sec2
Relation between Linear Motion and Angular Motion
Conclusion
We have discussed Kinematics of motion, and the displacement, velocity and acceleration in both the linear motion and the angular motion. If you have any further thoughts on this topic, let us know in the comment section below.

We teach these concepts to our high school physics students.
Yes, I do agree, we discuss both the basics and the advanced topics too. Thanks for your comment. Happy reading.