How to calculate Minimum starting speed of Centrifugal Pump?

The hydraulic machines, which convert the hydraulic energy into mechanical energy, are called turbines while the hydraulic machines which convert mechanical energy into hydraulic energy are called pumps. Hydraulic energy is in the form of pressure energy. If the mechanical energy is converted into pressure energy by means of centrifugal force acting on the fluid, the hydraulic machine is called a centrifugal pump. In the previous articles, we discussed how to calculate the Work done, Heads, And Efficiencies of a Centrifugal Pump. In this article Let us see how to calculate the Minimum starting speed of Centrifugal Pump.

Centrifugal Pump

As we mentioned Centrifugal Pump converts the mechanical energy into pressure energy by means of centrifugal force acting on the fluid.

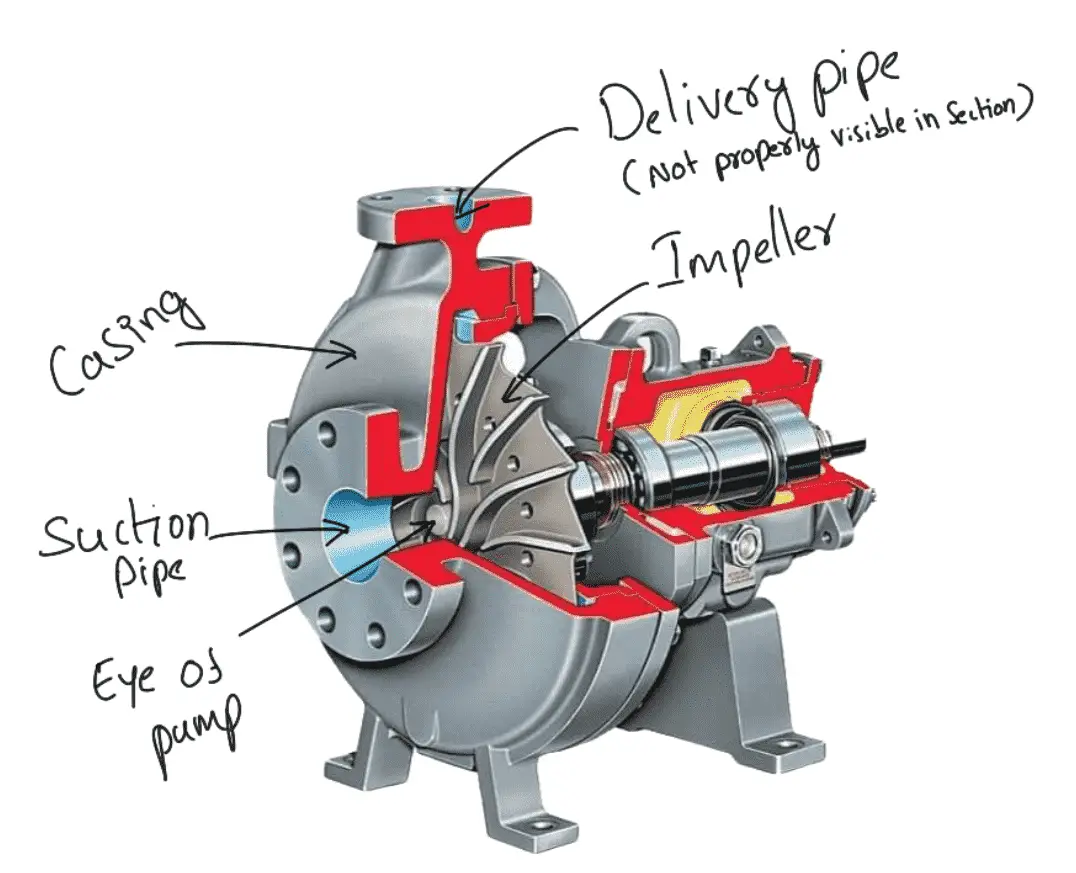
• The centrifugal pump acts as a reverse of an inward radial flow reaction turbine.
• This means that the flow in centrifugal pumps is in the radial outward directions.
• The centrifugal pump works on the principle of forced vortex flow.
• This means that when a certain mass of liquid is rotated by an external torque, the rise in pressure head of the rotating liquid takes place.
• The rise in pressure head at any point of the rotating liquid is proportional to the square of the tangential velocity of the liquid at that point.
• Thus at the outlet of the impeller, where the radius is more, the rise in pressure head will be more and the liquid will be discharged at the outlet with a high-pressure head.
• Due to this high-pressure head, the liquid can be lifted to a high level.

Minimum starting speed of Centrifugal Pump

If the pressure rise in the impeller is more than or equal to the manometric head (Hm), the centrifugal pump will start delivering water. Otherwise, the pump will not discharge any water, though the impeller is rotating. When the impeller is rotating, the water in contact with the impeller is also rotating. This is the case of the forced vortex.

In the case of the forced vortex, the centrifugal head or head due to pressure rise in the impeller

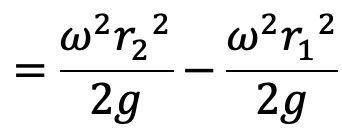
where
ωr₂ = Tangential velocity of the impeller at outlet = u₂
ωr₁ = Tangential velocity of the impeller at inlet = u₁

Head due to pressure rise in impeller

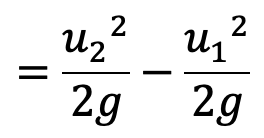
The flow of water will commence only if the Head due to pressure rise in the impeller ≥ H_m (or)

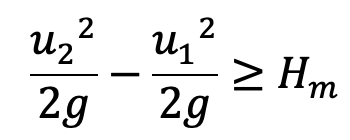
For minimum speed, we must have

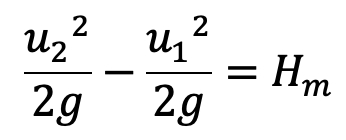
…. Equation (a)

from the equation Manometric Efficiency relation, we have

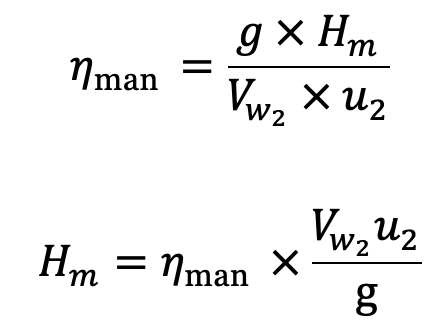
Substituting this value of H_m in the equation the above equation, we get

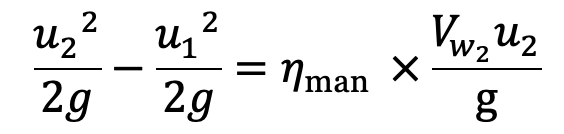
….. Equation(b)

We know

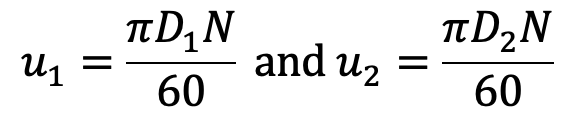
Substituting the values of u₂ and u₁, in the equation (b)

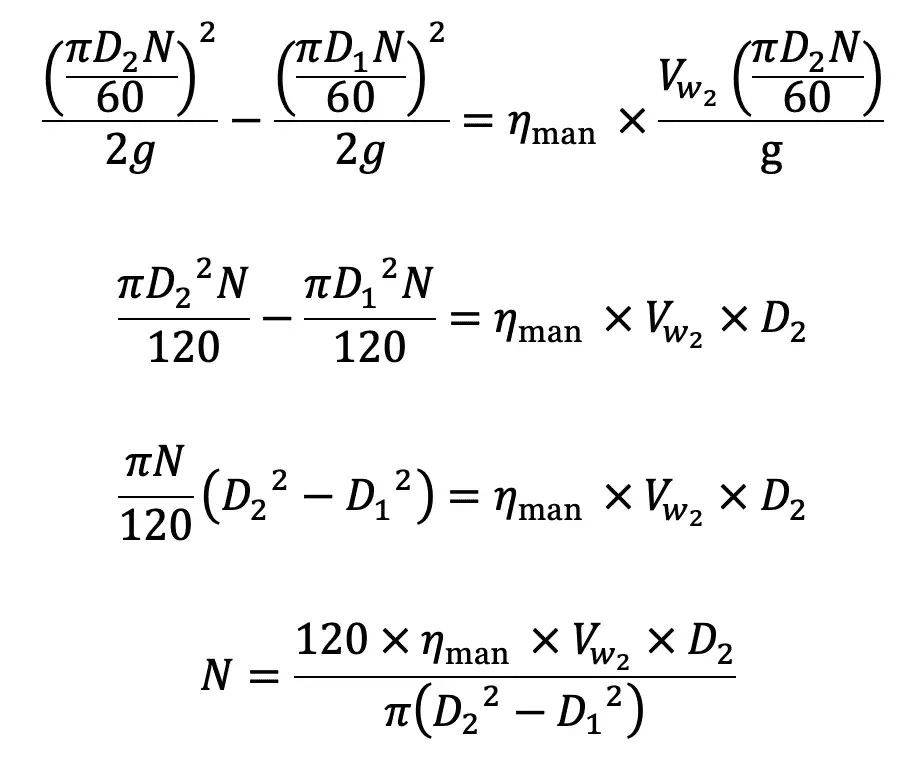
….. Equation (c)

Equation (b) gives the minimum starting speed of the centrifugal pump.

Let us solve an example problem to understand how to calculate the Minimum starting speed of Centrifugal Pump.

Example Problems on Minimum starting speed of Centrifugal Pump

Problem Statement: The diameters of an impeller of a centrifugal pump at the inlet and outlet are 30 cm and 60 cm respectively. Determine the minimum starting speed of the pump if it works against a head of 30m.

Answer:

Given data
Diameter of impeller at inlet D1 = 30 cm = 0.30m
Diameter of impeller at outlet D2 = 60 cm = 0.60 m
Head H_m = 30 cm
Let the minimum starting speed = N
Using equation (a) for minimum speed

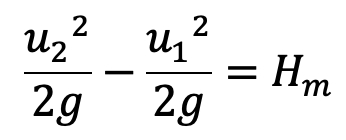
Where

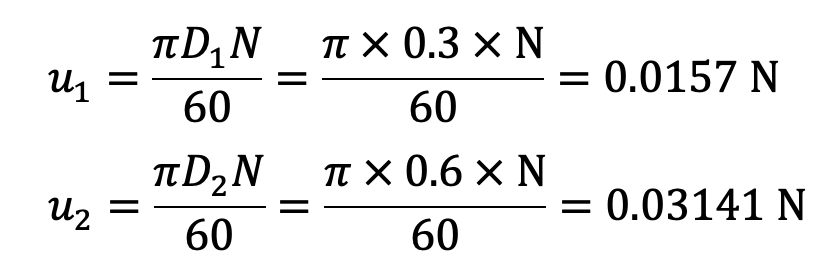
Let us substitute these in the above equation we get the Minimum starting speed of Centrifugal Pump

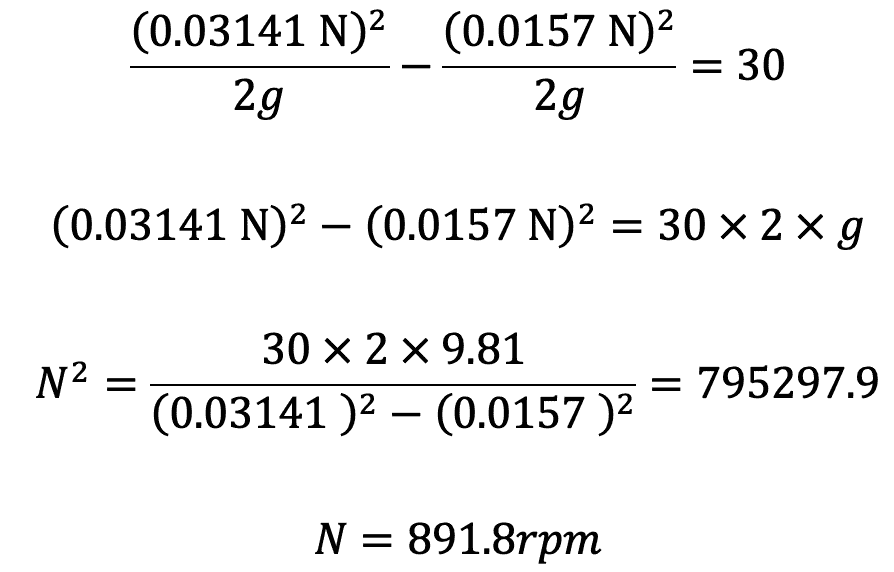
The Minimum starting speed of Centrifugal Pump is 892 rpm if it works against a head of 30m.

Would you like to solve an example problem by yourself?

Problem Statement: The diameters of an impeller of a centrifugal pump at inlet and outlet are 30 cm and 60 cm respectively. The velocity of flow at outlet is 2.0 m/s and the vanes are set back at an angle of 45° at the outlet. Determine the minimum starting speed of the pump if the manometric efficiency is 70%.

Solve it and let us know the answer in the comment section below. We will help you if you face any issues.