A truss is a structure made up of slender members or beams pin-connected at ends and is capable of taking loads at joints. Truss is a rigid structure. They are used as roof trusses to support sloping roofs and as bridge trusses to support the deck. In many machines, steel trusses are used. Transmission towers are also examples of trusses. In the case of wooden trusses, the ends are connected by making suitable joints or by nailing and bolting whereas in steel trusses ends are connected by bolting or welding. The trusses are also known as ‘pin-jointed frames’. Let us discuss more details on Trusses and see how we can calculate all forces in Truss.

A truss in which all the members lie in a single plane is called a plane truss. In such trusses loads act in the plane of the truss only. Roof trusses and bridge trusses can be considered plane trusses.
If all the members of a truss do not lie in a single plane, then it is called a space truss. Tripod and transmission towers are examples of space trusses.
The analysis of plane trusses is discussed below.
What is a Perfect Truss?
A pin-jointed truss that has got just a sufficient number of members to resist the loads without undergoing appreciable deformation in shape is called a perfect truss.
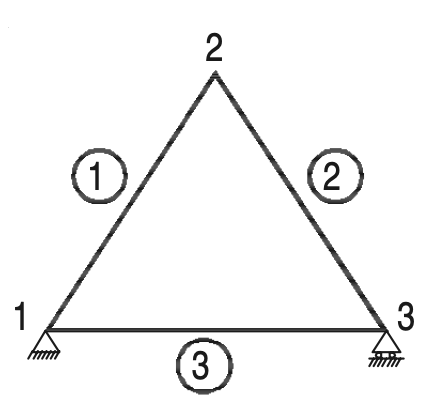
A triangular truss is the simplest perfect truss and it has three joints and three members as shown in the below figure.
Perfect trusses with four and five joints are shown in Figures (a) and (b) respectively.
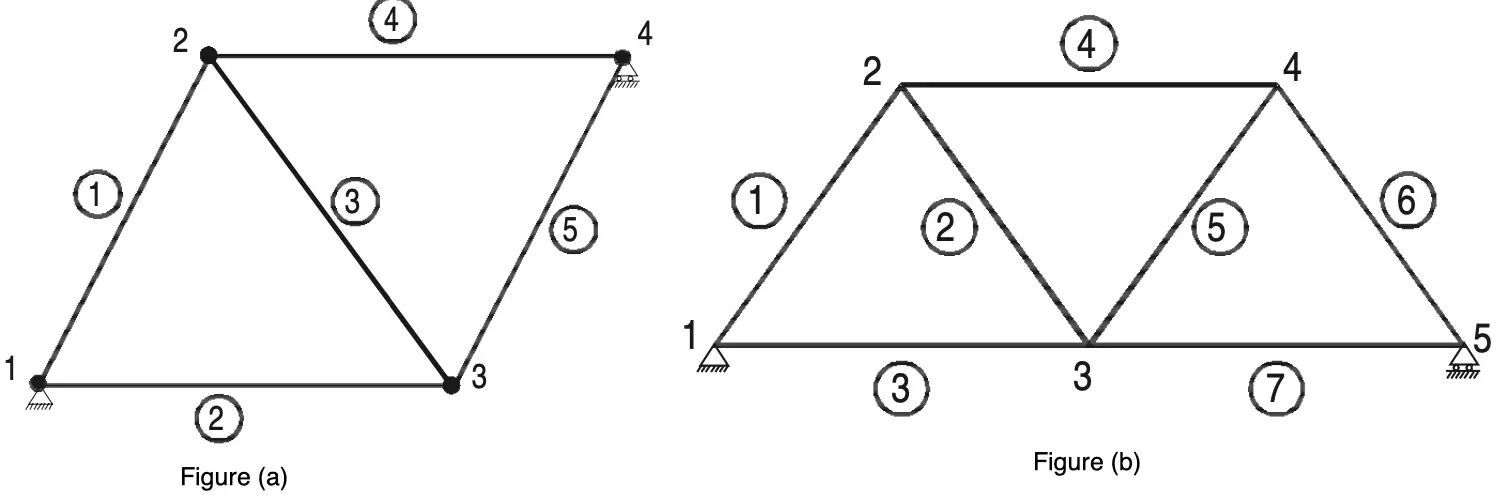
It may be observed that to increase one joint in a perfect truss, two more members are required.
Hence the following expression may be written down as the relationship between a number of joints j, and the number of members m, in a perfect truss.
m = 2j – 3
However, the above equation gives only a necessary, but not sufficient condition of a perfect truss. For example, the two trusses are shown in Figures (a) and (b) have the same number of members and joints.
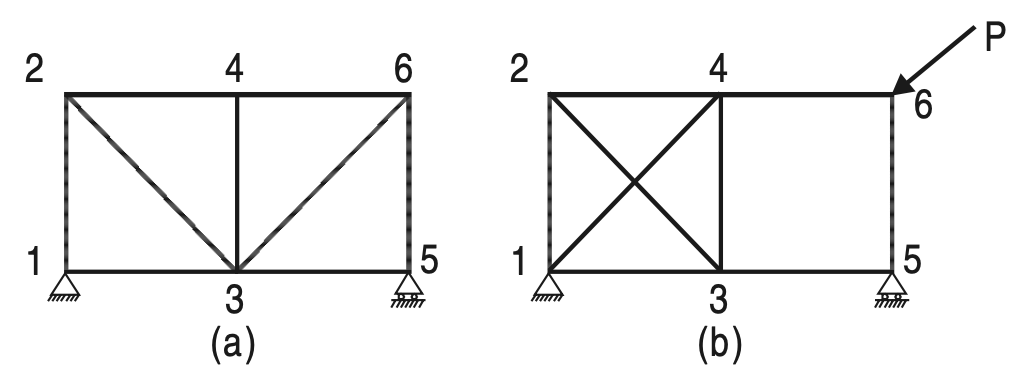
(b) Not a Perfect tress due to changing shape on applying load
The truss is shown in Figure (a) is perfect whereas the one shown in Figure (b) is not capable of retaining its shape if loaded at the joint marked 6. Therefore, the only necessary and sufficient condition of a perfect truss is that it should retain its shape when the load is applied at any joint in any direction.
What is a Deficient Truss?
A truss is said to be deficient if the number of members in it is less than that required for a perfect truss. Such trusses cannot retain their shape when loaded. A deficient truss is shown in the below figure.
What is a Redundant Truss?
A truss is said to be redundant if the number of members in it is more than that required in a perfect truss. Such trusses cannot be analyzed by making use of the equations of equilibrium alone.
Thus, a redundant truss is statically indeterminate. Each extra member adds one degree of indeterminacy. For the analysis of such members, the consistency of deformations is to be considered. The truss shown below is a typical redundant truss. In this truss one diagonal member in each panel is extra. Hence it is a two-degree redundant truss.
Let us only consider the perfect Truss for the analysis and let us see how we can calculate all the forces in Truss.
Assumptions for Analysis of Truss
In the theory that is going to be developed in this chapter, the following assumptions are made:
(1) The ends of the members are pin-connected (hinged)
(2) The loads act only at the joints
(3) Self-weights of the members are negligible
(4) Cross-section of the members is uniform
If at all the cross-section varies, the center of gravity of the section is assumed to be located along the same longitudinal line.
In reality, the members are connected by bolting, riveting, or by welding. No special care is taken to ensure perfect pin-connections. However, experiments have shown that assuming pin-connected ends is quite satisfactory since the members used are slender.
Nature Of Forces In Truss Members
The members of a truss are subjected to either tensile or compressive forces. A typical truss ABCDE loaded at joint E is shown in the below figure (a).
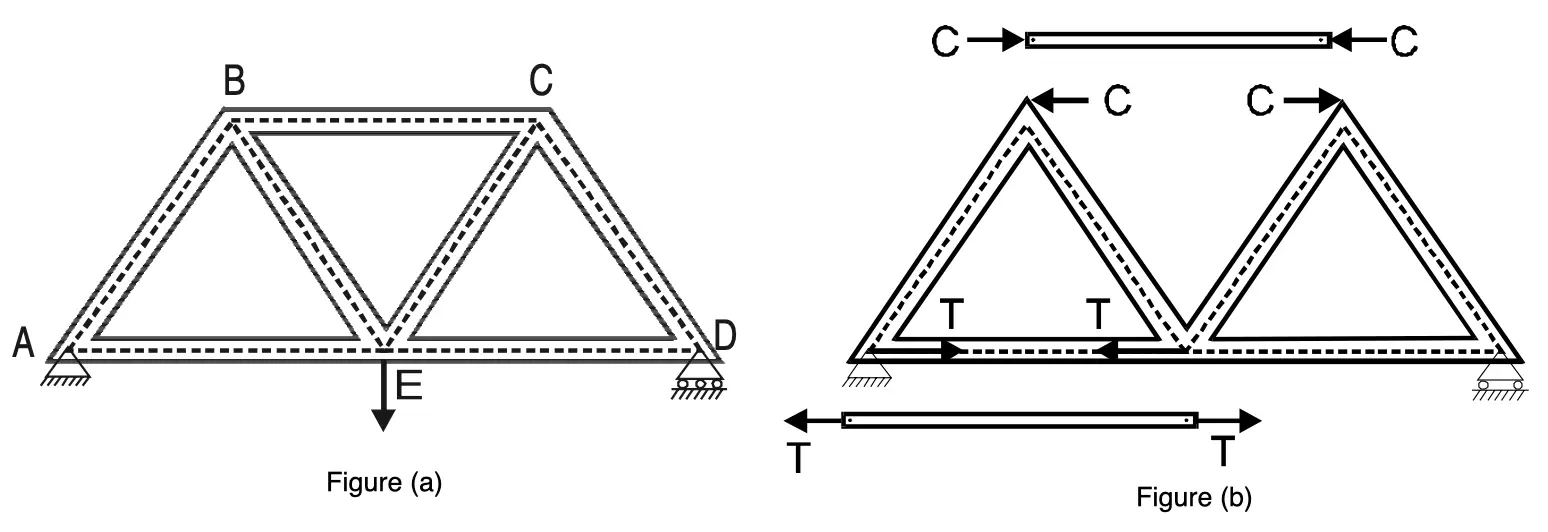
The member BC is subjected to compressive force C as shown in the above figure (b). The effect of this force on joint B (or C) is equal and opposite to the force C as shown in figure (b).
The member AE is subjected to tensile force T. Its effect on joints A and E are as shown in Figure (b) above. In the analysis of the frame, we mark the forces on the joints, instead of the forces in the members as shown in Figure below.
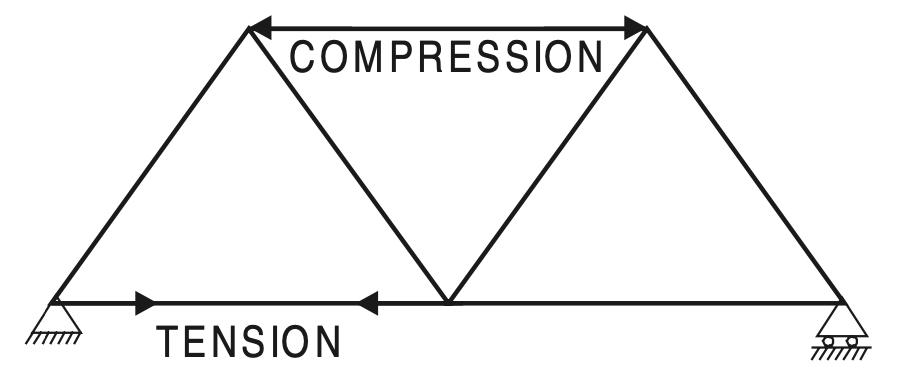
It may be noted that compressive force in a member is represented in a figure by two arrows going away from each other and a tensile force by two arrows coming towards each other. This is quite logical considering the fact that the markings on the members represent the internal reactive forces developed which are opposite in direction to the applied forces.
Methods Of Analysis
The following three methods are available for the analysis of pin-connected frames called Trusses.
(a) Method of joints
(b) Method of section
(c) Graphical method.
let us discuss how we can analyze the Truss with help of the method of Joints.
Calculating Forces in Truss by Method Of Joints
- At each joint the forces in the member’s meeting and the loads acting, if any, constitute a system of concurrent forces.
- Hence, two independent equations of equilibrium can be formed at each joint.
- First, a joint is selected where there are only two unknown forces.
- Many times such a joint can be identified only after finding the reaction at the support by considering the equilibrium of the entire frame.
- Then making use of the two equations of equilibrium at that joint the two unknown forces are found.
- Then, the next joint is selected for analysis where there are now only two unknown forces.
- Thus, the analysis proceeds from joints to joint to find the forces in all the members.
- It may be noted that if there are j number of joints, 2j number of the equations can be formed.
- There will be three reactions in a general determinate truss.
- The force in each member is unknown.
- Hence, if there are m number of members, the total number of unknowns will be m+3.
A problem can be analyzed if there are as many equations as there are unknowns.
Hence, a frame analysis problem is determinate if: 2j = m + 3
This equation is the same as the Equation above mentioned relation of a number of joints and number of members which was derived on the consideration of a perfect frame.
Hence, a perfect frame is determined.
- If m > 2j – 3, then the number of unknowns is more than the number of equations. Hence, a redundant frame is indeterminate.
- If m < 2j – 3, then the number of equations is more than the number of unknowns.
Since a set of solutions can satisfy such equations, it shows the instability of the structure. Hence, a deficient frame is not stable.
Let us explore this method of joints with help of example problems.
Example Problems to calculate all Forces in Truss with help of Method Of Joints
Problem Statement 1: Find the forces in all the members of the truss shown in the below figure. Please Tabulate the results.
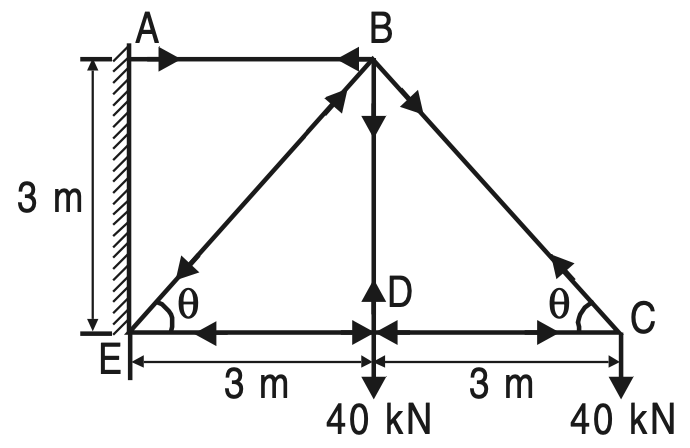
Answer:
Step by Step Procedure to calculate all Forces in a Truss with help of Methods of Joints
Step 1: Determine the inclinations of all inclined members.
In this case tan θ = 3/3 = 1
∴ θ = 45°
Step 2: Look for a joint at which there are only two unknowns. If such a joint is not available,
determine the reactions at the supports, and then at the supports, these unknowns may reduce to only two.
Now at joints C, there are only two unknowns, i.e., forces in members CB and CD, say FCB and FCD.
Note: Usually in cantilever type frames, we find such joints without the need to find reactions.
Step 3: Now there are two equations of equilibrium for the forces meeting at the joint and two unknown forces. Hence, the unknown forces can be determined.
At joint C as shown in the below figure ∑V = 0 condition shows that the force FCB should act away from
the joint C so that its vertical component balances the vertical downward load at C.
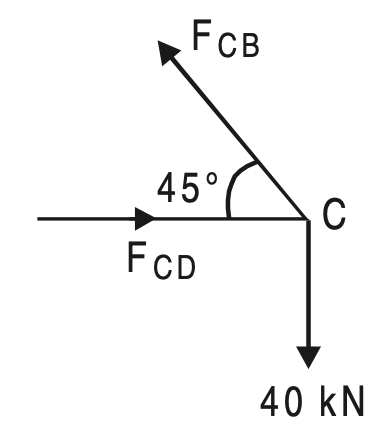
FCB sin 45° = 40
∴ FCB = 40 √2 kN
Now ∑H = 0 indicates that FCD should act towards C.
FCD – FCB cos 45° = 0
FCD = FCB cos 45°
FCD = 40 √2 × (1/√2) ×
FCD = 40 kN
Note: If the assumed direction of the unknown force is opposite, the value will be negative. Then reverse the direction and proceed.
Step 4: On the diagram of the truss, mark arrows on the members near the joint analyzed to indicate the forces on the joint. At the other end, mark the arrows in the reverse direction.
In the present case, near joint C, the arrows are marked on the member’s CB and CD to indicate forces FCB and FCD directions as found in the analysis of joint C.
Then reversed directions are marked in the members CB and CD near joints B and D, respectively.
Step 5: Look for the next joint where there are only two unknown forces and analyze that joint.
In this case, there are only two unknown forces at the joint D, as shown in the below figure.
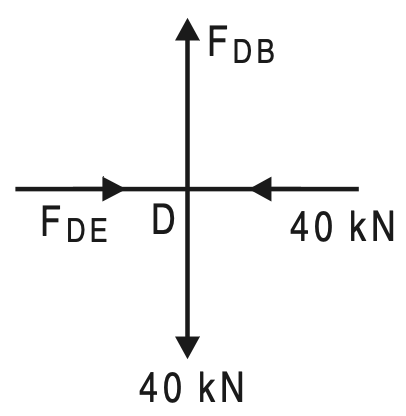
∑V = 0
FDB = 40 kN
∑ H = 0
FDE = 40 kN
Step 6: Repeat steps 4 and 5 till forces in all the members are found.
In the present case, after marking the forces in the members DB and DE, we find that analysis of joint B can be taken up. Referring to the below figure.
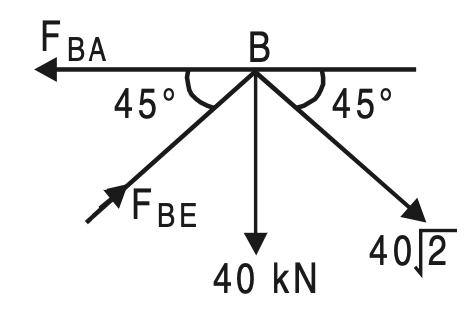
∑ V = 0, gives
FBE sin 45° – 40 – 40 √2 × sin 45° = 0
∴ FBE = 80 √2 kN
∑ H = 0
FBA – FBE cos 45° – 40√2 × cos 45° = 0
FBA = 80 √2 × (1/√2) + 40 √2× (1/√2)
∴ FBA = 120 kN
The directions of these forces are marked on the diagram.
Now the analysis is complete since the forces in all the members are determined.
Step 7: Determine the nature of forces in each member and tabulate the results. Note that if the arrow marks on a member are towards each other, then the member is in tension and if the arrow marks are away from each other, the member is in compression.

In this case,
| Member | Magnitude of Force in kN | Nature |
| AB | 120 | Tension |
| BC | 40√2 | Tension |
| CD | 40 | Compression |
| DE | 40 | Compression |
| BE | 80 √2 | Compression |
| BD | 40 | Tension |
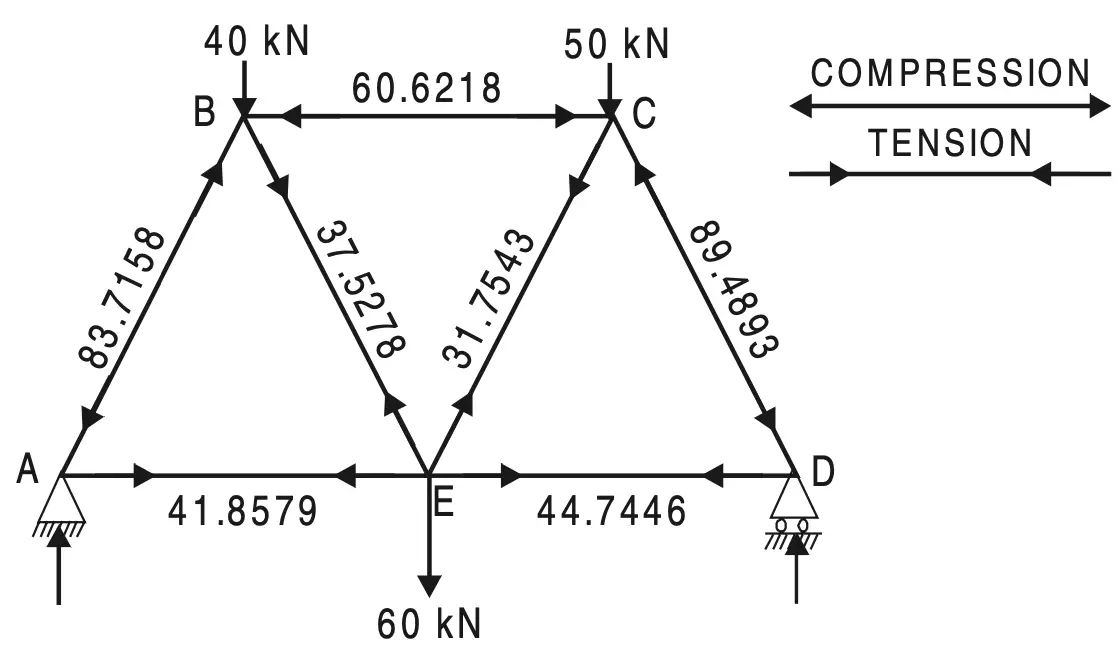
Problem Statement 2: Determine the forces in all the members of the truss shown in the below figure and indicate the magnitude and nature of forces on the diagram of the truss. All inclined members are at 60° to horizontal and the length of each member is 2 m.
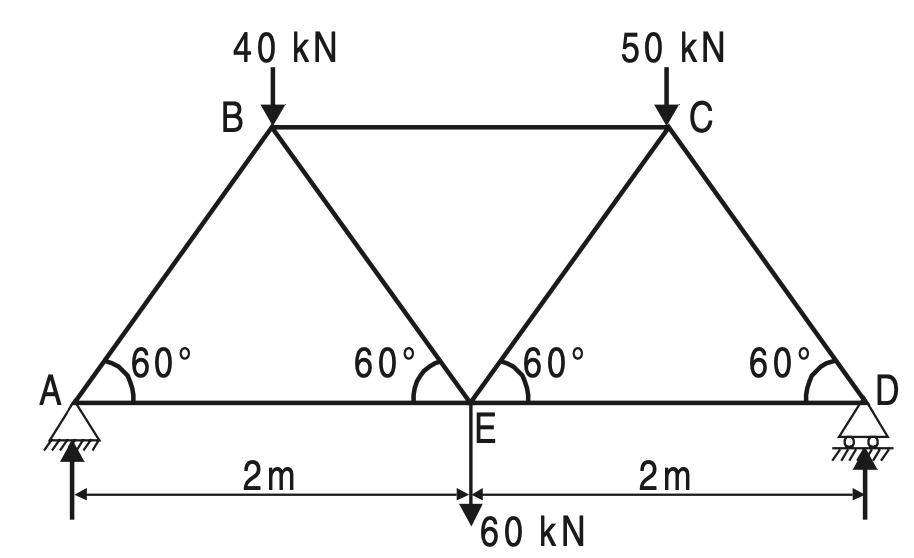
Solution:
Now, we cannot find a joint with only two unknown forces without finding reactions.
Consider the equilibrium of the entire frame.
∑ M A = 0, gives
RD × 4 – 40 × 1 – 60 × 2 – 50 × 3 = 0
∴ RD = 77.5 kN
∑ H = 0, gives
∴ HA = 0
∴ Reaction at A is vertical only
∑V = 0, gives
RA + 77.5 = 40 + 60 + 50
∴ RA = 72.5 kN
Joint A:
∑V = 0, gives
FAB sin 60° = RA = 72.5
FAB = 83.7158 kN (Compression)
∑ H = 0, gives
FAE – 83.7158 cos 60° = 0
FAE = 41.8579 kN (Tension)
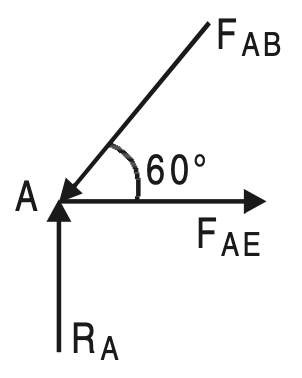
Joint D:
∑V = 0, gives
FDC sin 60° = RD = 77.5
∴ FDC = 89.4893 kN (Compression)
∑ H = 0, gives
FDE – 87.4893 cos 60° = 0
∴ FDE = 44.7446 kN (Tension)
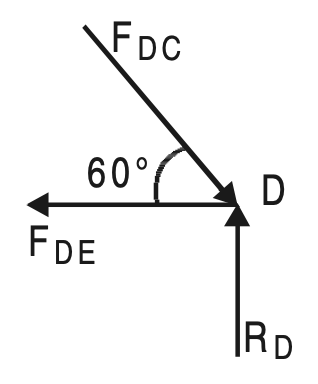
Joint B:
∑V = 0, gives
FBE sin 60° – FAB sin 60° + 40 = 0
FBE = (72.5- 40)/ sin60°
∴ FBE = 37 .5278 (Tension)
∑ H = 0, gives
FBC – FAB cos 60° – FBE cos 60° = 0
FBC = (83.7158 + 37.5274) × 0.5
FBC = 60.6218 kN (Compression)
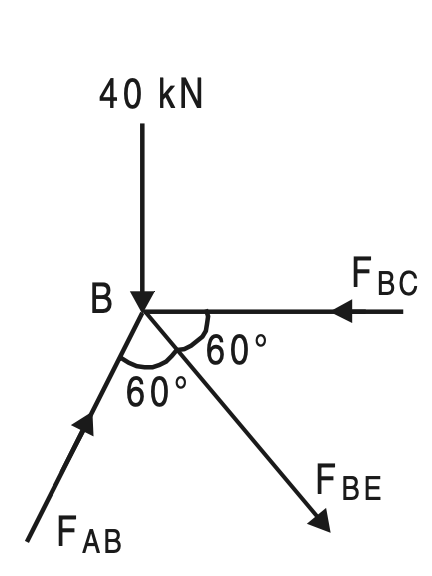
Joint C:
∑V = 0, gives
FCE sin 60° + 50 – FDC sin 60° = 0
FCE = (77.5- 50)/ sin 60
FCE = 31.7543 kN (Tension)
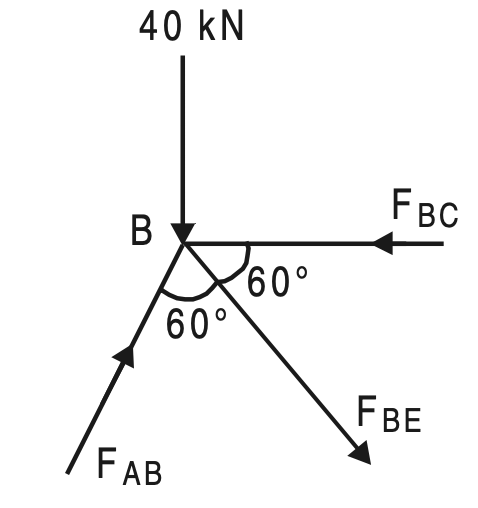
Now the forces in all the members are known. If joint E is analyzed it will give the check for
the analysis. The results are shown on the diagram of the truss in the below figure.
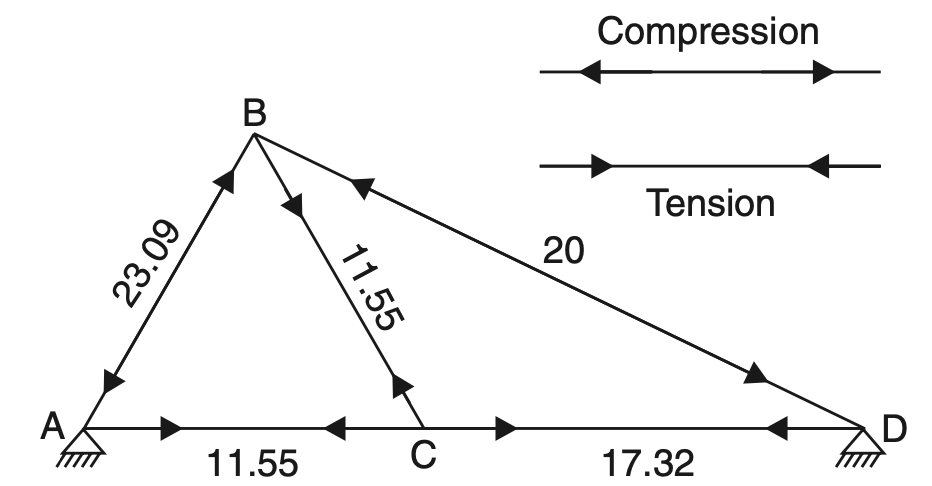
Problem Statement 3: Determine the forces in all the members of the truss shown in the below figure.
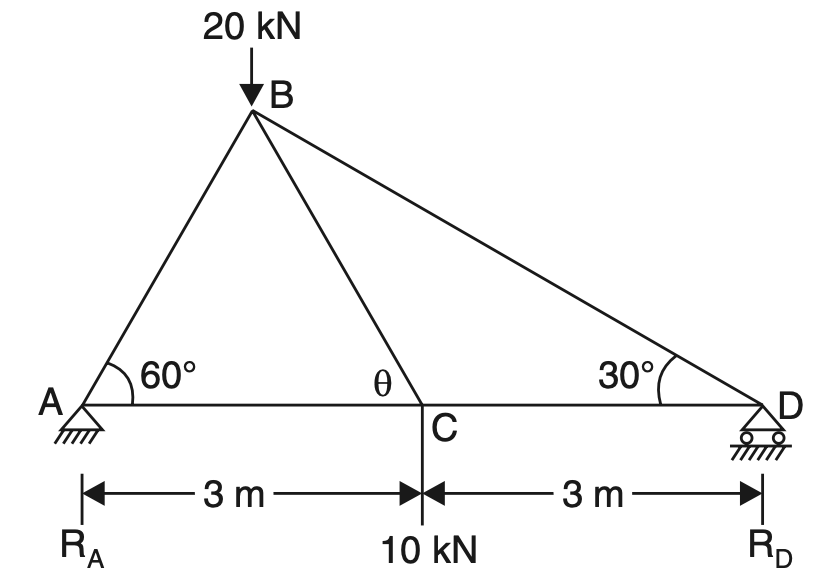
Solution:
AB = 6 sin 60° = 3 m;
BD = 6 cos 30° = 23.09 m
In ∆ABC, AC = AB = 3 m
∴ ∠ABC = ∠BCA = say θ
∴ 2θ = 180 – 60 = 120
θ = 60°
Taking moment about A, we get
RD × 6 – 10 × 3 – 20 × 3 cos 60° = 0
RD = 10 kN
∴ RA = 20 + 10 – 10 = 20 kN
[Note: H4 = 0]
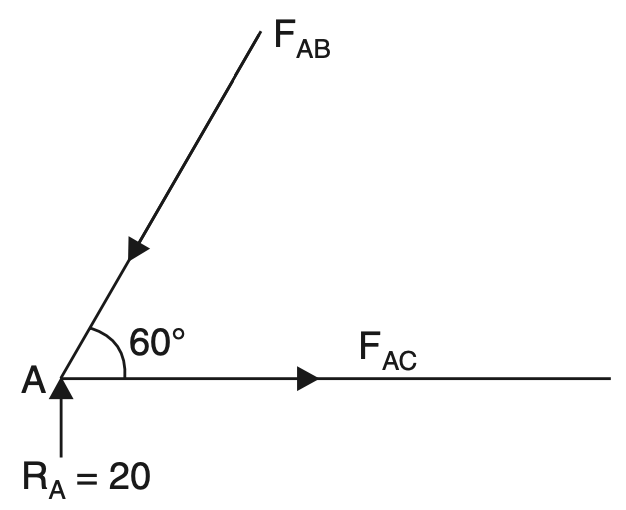
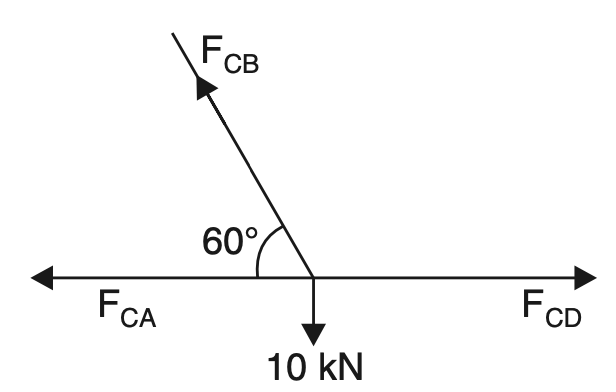
Joint A: Referring to above figure
ΣV = 0, gives
20 – FAB sin 60° = 0
∴ FAB = 23.09 kN [Comp.]
ΣH = 0, gives
FAC – FAB cos 60° = 0
∴ FAC = 23.09 cos 60° = 11.55 kN [Tensile]
Joint D:
ΣV = 0, gives
FDB sin 30° = 10
FDB = 20 kN [Compression]
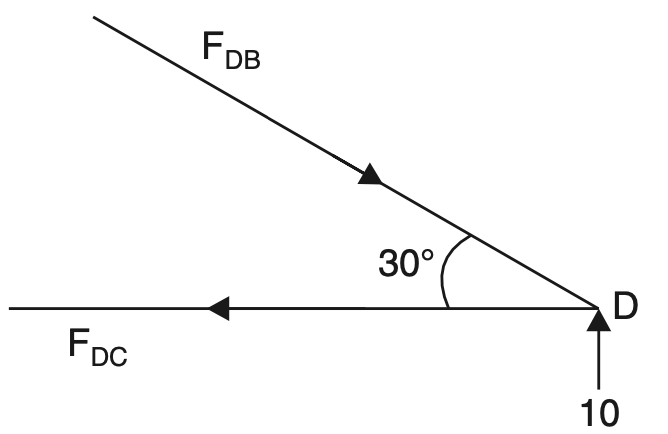
ΣH = 0, gives
– FDC + FDB cos 30° = 0
FDC = 20 cos 30°
FDC = 17.32 kN [Tensile]
Joint C:
ΣV = 0, gives
FCB sin 60° = 10
∴ FCB = 11.55 kN
check ΣH = 0, gives
FCB cos 60° = FCD – FCA = 17.32 – 11.55
∴ FCB = 11.55, Checked
Let us mark all these reaction forces in a single diagram.
Calculating Forces in Truss by Method Of Section
- In the method of the section, after determining the reactions, a section line is drawn passing through not more than three members in which forces are not known such that the frame is cut into two separate parts.
- Each part should be in equilibrium under the action of loads, reactions, and forces in the members that are cut by the section line.
- Equilibrium of any one of these two parts is considered and the unknown forces in the members cut by the section line are determined.
- The system of forces acting on either part of the truss constitutes a non-concurrent force system.
- Since there are only three independent equations of equilibrium, there should be only three unknown forces.
- Hence, in this method, it is an essential condition that the section line should pass through not more than three members in which forces are not known and it should separate the frame into two parts.
Thus, the method of the section is the application of nonconcurrent force system analysis whereas the method of joints, described in the previous article was the application of analysis of concurrent force
system.
Under the following two conditions the method of the section is preferred over the method of joints:
(1) In a large truss in which forces in only a few members are required
(2) In the situation where the method of joints fails to start/proceed with the analysis
Read more about the method here. We also discussed 3 example problems to calculate all forces in Truss with the Method of section.
Conclusion
We have discussed what is a perfect truss, a Redundance truss, and a deficient Truss. We have listed the assumptions that we make to analyze the Truss, also list the types of forces in truss members. We calculate all the forces in the truss given in 3 example problems successfully with help of the method of Joint. Let us know what do you think about this article in the comment section below.

Good day,An exelent artícul.
Thanks.