What is the Atkinson Cycle?

We have already discussed the Otto cycle in the previous article, This Atkinson Cycle is an extension cycle for the Otto cycle. Let’s discuss more on it.

Atkinson Cycle

In the Otto cycle, there are two Isentropic processes(Isentropic compression and the Isentropic Expansion), and two Constant volume Processes. The Isentropic Expansion of this Otto cycle is further allowed to proceed to the lowest cycle pressure in order to increase the work output. So that is why this Atkinson cycle is the extended Otto cycle.

In modern days, people are taking this Atkinson cycle advantage in building Hybrid Engines to make the engine a bit more efficient.

As we said above, Otto cycle has the following four processes.

• Suction or intake stroke,
• Compression stroke,
• Expansion or power stroke,
• Exhaust stroke

During the compression stroke, the inlet valve/port will be still opened for some time to decrease the compression ratio. Let’s say in Otto cycle the compression ratio is 10:1 then in the Atkinson Cycle, it can be less than that. For example, it is 8:1.

So why do we need to reduce the compression ratio for this cycle?

Because, whereas in Otto cycle during the expansion stroke after the combustion, the force on the piston will be more and while the piston reaches the bottom dead centre then the exhaust valves/ports will be opened, so the excess amount of energy will be lost.

To avoid this, Atkinson Cycle reduces the compression ratio to expand more in the expansion stroke to use the entire force on piston (due to high pressure generated in the combustion stroke) before the piston reaches to the bottom dead center.

So this means the Atkinson Cycle will always have the less/equivalent power than the Otto cycle. But the Thermal Efficiency of the Atkinson cycle is more than the Otto cycle.

Check out the P-V diagram and T-S diagram in the Atkinson Cycle

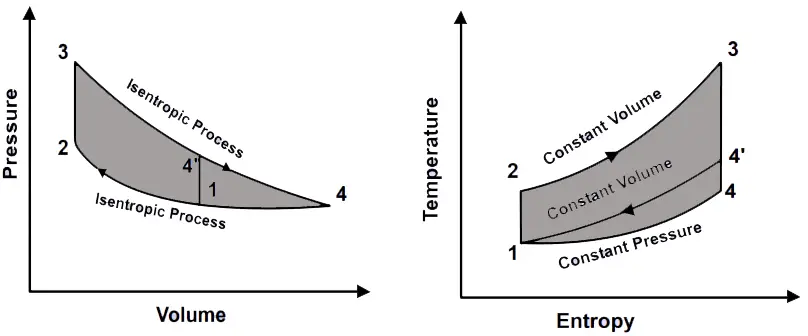
Otto Cycle P-V diagram and T-S diagram

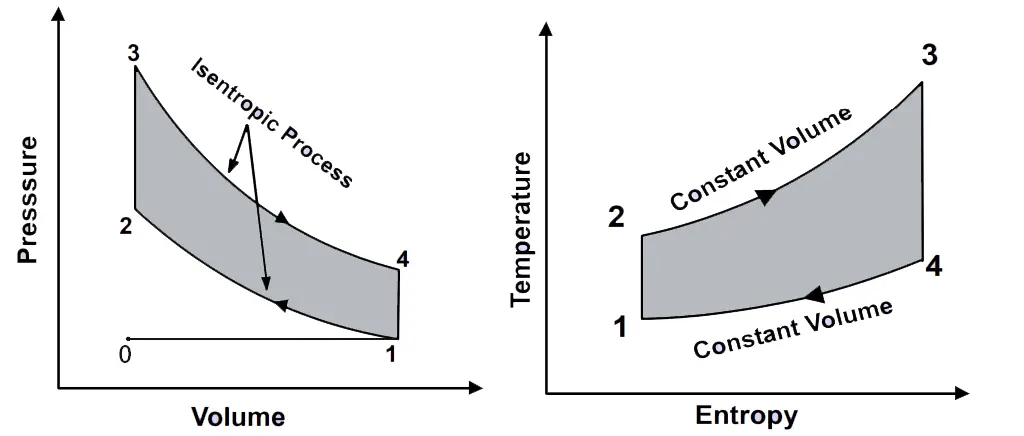
Thermal Efficiency of the Atkinson Cycle

Thermodynamically, efficiency of the Otto cycle is given by

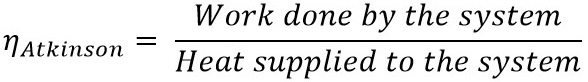
Work done by the system = Heat supplied (Q_S)- Heat rejected (Q_R)

W = Q_S – Q_R

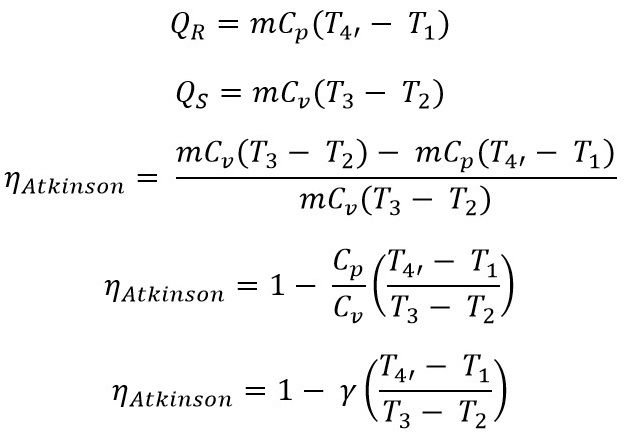
The Compression Ratio r = V₁/V₂ and the Expansion Ratio e = V_4’/V₃.

Now Considering the isotropic processes 1 → 2

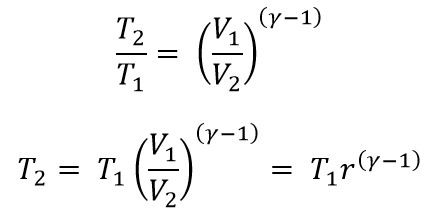
Considering the 2 → 3 process

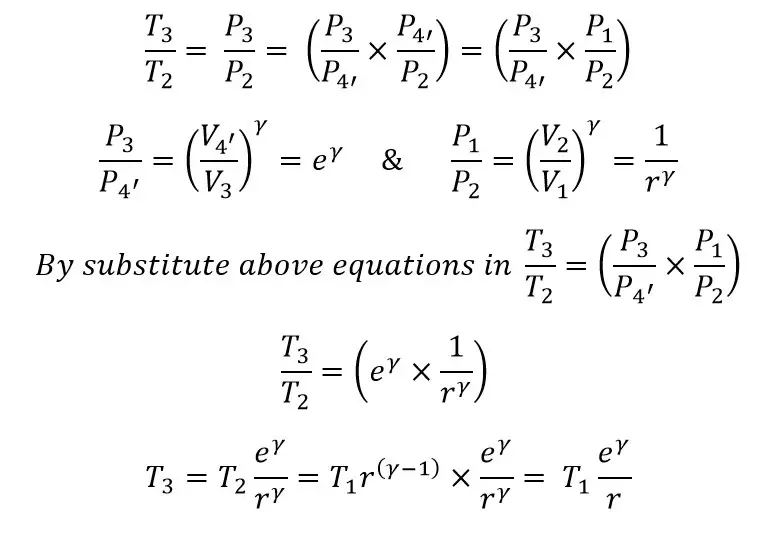
Considering the 3 → 4′ process

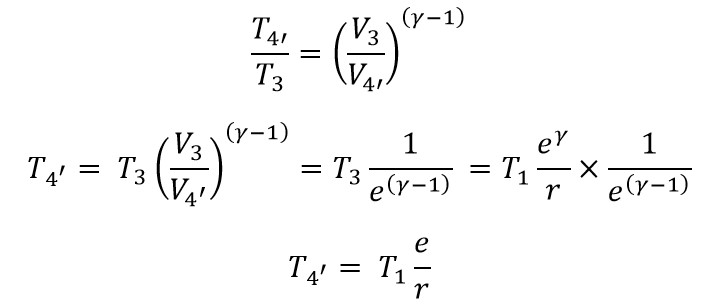
By substituting the T₂ ,T₃ ,T₄ in the above Efficiency formula

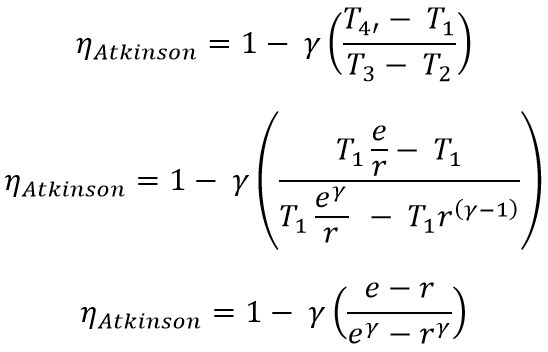
This is the Thermal Efficiency equation for the Atkinson Cycle. The thermal efficiency of the Atkinson cycle will be more than the Otto cycle. But the power output will be less than the Otto cycle.

Conclusion

We have discussed how the Atkinson Cycle is extended to get the maximum thermal efficiency from the engine and also we have derived the efficiency equation for the Atkinson Cycle. Please let us know your thoughts in the comment section below.